In figure, AB is a chord of the circle and AOC is its diameter such that ∠ACB = 50°. If AT is the tangent to the circle at the point A, then ∠BAT is equal to

Question

In figure, AB is a chord of the circle and AOC is its diameter such that ∠ ACB = 50°. If AT is the tangent to the circle at the point A, then ∠ BAT is equal to

Philoid · Accepted Answer

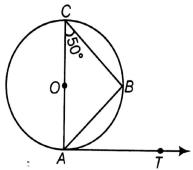
Given : A circle with center O and AC is its diameter and ∠ACB = 50° , Also AT is a tangent to the circle at point A
To Find : ∠BAT

We Have

∠CBA = 90° [ As angle in a semicircle is a right angle]

So, By angle sum property of a triangle

∠ACB + ∠CAB + ∠CBA = 180°
50° + ∠CAB + 90° = 180°

∠CAB = 40° [1]

Also

OA ⏊ AT [ As tangent to at any point on the circle is perpendicular to the radius through point of contact]

So, ∠OAT = 90°

∠OAT + ∠BAT = 90°

∠CAT + ∠BAT = 90°

40° + ∠BAT = 90° [ using 1]

∠BAT = 50°