In figure, if 0 is the center of a circle, PQ is a chord and the tangent PR at P makes an angle of 50° with PQ, then ∠POQ is equal to
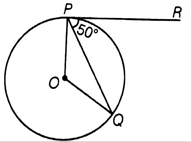
Given : OP is a radius and PR is a tangent in a circle with center O with ∠RPQ = 50°
To find : ∠POQ
Now, OP ⏊ PR [ As tangent to at any point on the circle is perpendicular to the radius through point of contact]
∠OPR = 90°
∠OPQ + ∠RPQ = 90°
∠OPQ + 50° = 90°
∠OPQ = 40°
In △POQ
OP = OQ [radii of same circle]
∠OQP = ∠OPQ = 40° [ angles opposite to equal sides are equal] [1]
In △OPQ By angle sum property of a triangle
∠OPQ + ∠OPQ + ∠POQ = 180°
40° + 40° + ∠POQ = 180°
∠POQ = 100°
8