True

Given : AB is a diameter of circle with center O and AC is a chord such that ∠BAC = 30° Also tangent at C intersects AB extends at D.

To prove : BC = BD

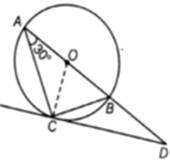
Proof :

OA = OC [radii of same circle]

∠OCA = ∠OAC = 30° [Angles opposite to equal sides are equal]

∠ACB = 90° [angle in a semicircle is a right angle.]

∠OCA + ∠OCB = 90°

30° + ∠OCB = 90°

∠OCB = 60° [1]

OC = OB [ radii of same circle]

∠OBC = ∠OCB = 60° [angles opposite to equal sides are equal]

Now, ∠OBC + ∠CBD = 180° [linear pair]

60 + ∠CBD = 180°

So, ∠CBD = 120° [2]

Also,

OC ⏊ CD [Tangent at a point on the circle is perpendicular to the radius through point of contact ]

∠OCD = 90°

∠OCB + ∠BCD = 90°

60 + ∠BCD = 90

∠BCD = 30° [3]

In △BCD

∠CBD + ∠BCD + ∠BDC = 180° [Angle sum property of triangle]

120° + 30° + ∠BDC = 180° [From 2 and 3]

∠BDC = 30° [4]

From [3] and [4]

∠BCD = ∠BDC = 30°

BC = BD [Sides opposite to equal angles are equal]

Hence Proved !