If from an external point B of a circle with center O, two tangents BC and BD are drawn such that ∠ DBC = 120°, prove that BC + BD = BO i.e., BO = 2 BC.

Question

Philoid · Accepted Answer

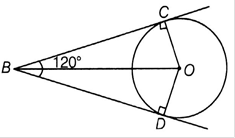
Given : BC and BD are two tangents drawn from a common external point to a circle with center O such that ∠DBC = 120°

To Prove : BC + BD = BO i.e. BO = 2BC

Proof :

In △OBC and △ODB

OC = OC [ Radii of same circle ]

OB = OB [Common]

BC = BD [Tangents drawn from an external point to a circle are equal ] [1]

△OBC ≅ △OBD [ By Side Side Criterion ]

∠CBO = ∠DBO [Corresponding parts of congruent triangles are equal ]

Also,

∠DBC = 120°

∠CBO + ∠DBO = 120°

∠CBO + ∠CBO = 120°

∠CBO = ∠DBO = 60°

OC ⏊BC [Tangent at a point on the circle is perpendicular to the radius through point of contact ]

Therefore,

OBC is a right-angled triangle at C

So we have,

BO = 2BC

From [1] we have

BC = BD

BC + BC = BC + BD

2BC = BC + BD

BO = BC + BD

Hence Proved .

If from an external point B of a circle with center O, two tangents BC and BD are drawn such that ∠DBC = 120°, prove that BC + BD = BO i.e., BO = 2 BC.