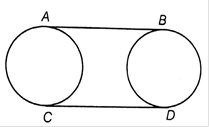
Given: AB and CD are two common tangents to two circles of equal radii .

To Prove: AB = CD

Construction: Join OA, OC, O’B and O’D

Proof:

Now, ∠OAB = 90° and ∠OCD = 90° as OA ⏊ AB and OC ⏊ CD

[tangent at any point of a circle is perpendicular to radius through the point of contact]

Thus, AC is a straight line.

Also,

∠O'BA = ∠O'DC = 90° [Tangent at a point on the circle is perpendicular to the radius through point of contact]

Thus, BD is Also a straight line.

So ABCD is a quadrilateral with Four sides as AB, BC, CD and AD

But as

∠A = ∠B = ∠C = ∠D = 90°

So, ABCD is a rectangle.

Hence, AB = CD [opposite sides of rectangle are equal]