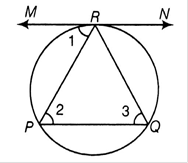
PQ is a chord in a circle with center O and MN is a tangent drawn at point R on the circle PQ is parallel to MN

To Prove : R bisects the arc PRQ i.e. arc PR = arc PQ

Proof :

∠1 = ∠2 [Alternate Interior angles]

∠1 = ∠3 [angle between tangent and chord is equal to angle made by chord in alternate segment]

So, we have

∠2 = ∠3

QR = PR [Sides opposite to equal angles are equal]

As the equal chords cuts equal arcs in a circle.

Arc PR = arc RQ

R bisects the arc PRQ .

Hence Proved