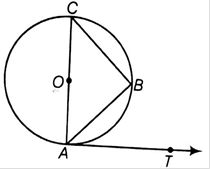
Given : A circle with center O and AC as a diameter and AB and BC as two chords also AT is a tangent at point A

To Prove : ∠BAT = ∠ACB

Proof :

∠ABC = 90° [Angle in a semicircle is a right angle]

In △ABC By angle sum property of triangle

∠ABC + ∠ BAC + ∠ACB = 180 °

∠ACB + 90° = 180° - ∠BAC

∠ACB = 90 - ∠BAC [1]

Now,

OA ⏊ AT [Tangent at a point on the circle is perpendicular to the radius through point of contact ]

∠OAT = ∠CAT = 90°

∠BAC + ∠BAT = 90°

∠BAT = 90° - ∠BAC [2]

From [1] and [2]

∠BAT = ∠ACB [Proved]