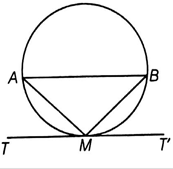
Let us draw a circle in which AMB is an arc and M is the mid-point of the arc AMB. Joined AM and MB. Also TT' is a tangent at point M on the circle.

To Prove : AB || TT'

Proof :

As M is the mid point of Arc AMB

Arc AM = Arc MB

AM = MB [As equal chords cuts equal arcs]

∠ABM = ∠BAM [Angles opposite to equal sides are equal] [1]

Now,

∠BMT' = ∠BAM [angle between tangent and the chord equals angle made by the chord in alternate segment] [2]

From [1] and [2]

∠ABM = ∠BMT'

So, AB || TT' [two lines are parallel if the interior alternate angles are equal]

Hence Proved !