If an isosceles ΔABC in which AB = AC = 6cm, is inscribed in a circle of radius 9 cm, find the area of the triangle.
Given : In a circle, ΔABC is inscribed in which AB = AC = 6 cm and radius of circle is 9 cm
To Find : Area of triangle ABC
Construction : Join OB and OC
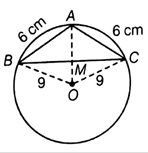
In △AOB and △AOC
OB = OC [Radii of same circle]
AO = AO [Common]
AB = AC = 6 cm [Given]
△AOB ≅ △AOC [By Side Side Side Criterion]
∠OAB = ∠OBC [Corresponding parts of congruent triangles are equal ]
Implies ∠MAB = ∠MBC
Now in △ABM and △AMC
AB = AC = 6 cm [Given]
AM = AM [Common]
∠MAB = ∠MBC [Proved Above]
△ABM ≅ △AMC [By Side Angle Side Criterion]
∠AMB = ∠AMC [Corresponding parts of congruent triangles are equal ]
Now,
∠AMB + ∠ AMC = 180°
∠AMB + ∠AMB = 180°
∠AMB = 180
∠AMB = 90°
We know that a perpendicular from center of circle bisects the chord.
So, OA is perpendicular bisector of BC.
Let OM = x
Then, AM = OA - OM = 9 - x
[ As OA = radius = 9 cm]
In right angled ΔAMC, By Pythagoras theorem
(AM)2 + (MC)2 = (AC)2
(9 - x)2 + (MC)2 = (6)2
81 + x2- 18x + (MC)2 = 36
(MC)2 = 18x - x2 - 45 [1]
In △OMC , By Pythagoras Theorem
(MC)2 + (OM)2 = (OC)2
18x - x2 - 45 + (x)2 = (9)2
18x - 45 = 81
18x = 36
x = 2
AM = 9 - x = 9 - 2 = 7 cm
In △AMC, By Pythagoras Theorem
(AM)2 + (MC)2 = (AC)2
(7)2+ (MC)2 = (6)2
49 + (MC)2= 36
(MC)2 = 25
MC = 5 cm
Now,
As MC = BM
BC = 2MC = 2(5) = 10 cm
And
![]()