The area of the square that can be inscribed in a circle of radius 8 cm is
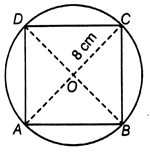
Let r be the radius of circle = OC = 8 cm.
∴ Diameter of the circle = AC = 2 × OC = 2 × 8 = 16 cm
Let a be the side of the square.
Now, according to the given condition,
Diagonal of square = Diameter of the circle.
Now in right angled triangle ACB,
(AC)2 = (AB)2 + (BC)2
(By Pythagoras theorem)
(16)2 = a2 + a2
⇒ 256 = 2a2
⇒ a2 = 128
∴ Area of the square = a2 = 128 cm2
9