Sides of a triangular field are 15 m, 16 m, and 17m. with the three corners of the field a cow, a buffalo and a horse are tied separately with ropes of length 7m each to graze in the field.

Question

Philoid · Accepted Answer

Sides of the triangle are 15 m, 16 m, and 17 m.

Now, perimeter of the triangle = (15+16+17) m = 48 m

∴ Semi-perimeter of the triangle = s = 48/2 = 24 m

By Heron’s formula, Area of the triangle =

(where a, b, c are the sides of triangle)

=

= 109.982 m²

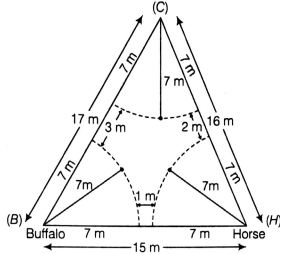
Let B, C and H be the corners of the triangle on which buffalo, cow and horse are tied respectively with ropes of

7 m each.

So, the area grazed by each animal will be in the form of a sector.

∴ Radius of each sector = r = 7 m

Let x, y, z be the angles at corners B, C, H respectively.

∴ Area of sector with central angle x =

Area of sector with central angle y =

Area of sector with central angle z =

Area of field not grazed by the animals = Area of triangle – (area of the three sectors)

=

= 109.892 – 77 = 32.982 cm²

Sides of a triangular field are 15 m, 16 m, and 17m. with the three corners of the field a cow, a buffalo and a horse are tied separately with ropes of length 7m each to graze in the field.Find the area of the field which cannot be grazed by the three animals.

Sides of a triangular field are 15 m, 16 m, and 17m. with the three corners of the field a cow, a buffalo and a horse are tied separately with ropes of length 7m each to graze in the field.
Find the area of the field which cannot be grazed by the three animals.