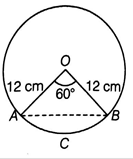
Radius of the circle = r = 12 cm

∴ OA = OB = 12 cm

∠ AOB = 60° (given)

Since triangle OAB is an isosceles triangle, ∴ ∠ OAB = ∠ OBA = θ (say)

Also, Sum of interior angles of a triangle is 180°,

∴ θ + θ + 60° = 180°

⇒2θ = 120° ⇒ θ = 60°

Thus, the triangle AOB is an equilateral triangle.

∴ AB = OA = OB = 12 cm

Area of the triangle AOB = × a² ,

where a is the side of the triangle.

× (12)²

= (√3/16) ×144

=

= 62.354 cm²

Now, Central angle of the sector AOBCA = = 60° = = (π/3) radians

Thus, area of the sector AOBCA =

= = 75.36 cm²

Now, Area of the segment ABCA = Area of the sector AOBCA – Area of the triangle AOB

= (75.36 – 62.354) cm² = 13.006 cm²