In figure, ABCD is a trapezium with AB||DC. AB = 18 cm, DC = 32 cm and distance between AB and DC = 14 cm. If arcs of equal radii 7 cm with centers A, B, C and D have been drawn, then find the area of the shaded region of the figure.
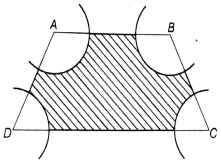
AB = 18 cm, DC = 32 cm
Distance between AB and DC = Height = 14 cm
Now, Area of the trapezium = (1/2) × (Sum of parallel sides) × Height
= (1/2) × (18+32) × 14 = 350cm2
As AB ∥ DC, ∴ ∠ A +∠ D = 180°
and ∠ B +∠ C = 180°
Also, radius of each arc = 7 cm
Therefore,
Area of the sector with central angle A = (1/2) × (∠A/180) × π × r2
Area of the sector with central angle D = (1/2) × (∠D/180) × π × r2
Area of the sector with central angle B (1/2) × (∠B/180) × π × r2
Area of the sector with central angle C = (1/2) × (∠C/180) × π × r2
Total area of the sectors = ![]()
![]()
![]()
= 77 + 77 = 154
∴ Area of shaded region = Area of trapezium – (Total area of sectors)
= 350 – 154 = 196 cm2
Hence, the required area of shaded region is 196 cm2.