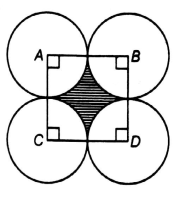
The four circles are placed in such a way that each piece touches the other two pieces.

So, by joining the centers of the circles by a line segment, we get a square ABDC with sides as,

AB = BD = DC = CA = 2(Radius) = 2(7) cm = 14 cm

Now, Area of the square = (Side)² = (14)² = 196 cm²

Since, ABDC is a square, ∴ each angle has a measure of 90°.

∴ ∠ A = ∠ B = ∠ D = ∠ C = 90° = π/2 radians = θ (say)

Also, Radius of each sector = 7 cm

Thus,

Area of the sector with central angle A = (1/2)r²θ

=

=

= (77/2) cm²

Since the central angles and the radius of each sector are same, therefore area of each sector is 77/2 cm²

∴ Area of the shaded portion = Area of square – Area of the four sectors

= 196 – 154

= 42 cm²

Hence, required area of the portion enclosed between these pieces is 42 cm².