On a square cardboard sheet of area 784 cm2, four congruent circular plates of maximum size are placed such that each circular plate touches the other two plates and each side of the square sheet is tangent to two circular plates. Find the area of the square sheet not covered by the circular plates.
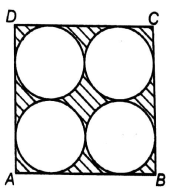
Since, Area of the square = 784 cm2
∴ Side of the square = √Area = √784 = 28 cm
Since the four circular plates are congruent, therefore diameter of each circular plate = 28/2 = 14 cm
∴ Radius of each circular plate = 7 cm
Area of the sheet not covered by plates =
Area of the square – Area of the four circular plates
Since all four circular plates are congruent, therefore area of all four plates will be equal.
∴ Are of one circular plate = πr2 = ![]() = 154 cm2
= 154 cm2
So, Area of four plates = 4×154 = 616 cm2
Area of the sheet not covered by plates = 784 – 616 = 168 cm2