Given,

In ∆PQR

PS = QS + RS ……(i)

In ∆PSR

PS = RS ….. [from Equation (i)]

⇒ ∠1 = ∠2 Equation ….(ii)

Similarly,

In ∆RSQ,

⇒ ∠3 = ∠4 Equation……(iii)

[Corresponding angles of equal sides are equal]

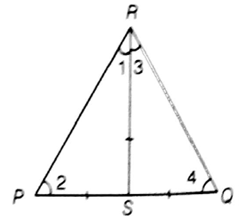
[By using Equations (ii) and (iii)]

Now in,

∆PQR, sum of angles = 180°

⇒ ∠P + ∠Q + ∠R = 180°

⇒ ∠2 + ∠4 + ∠1 + ∠3 = 180°

⇒ ∠1 + ∠3 + ∠1 + ∠3 = 180°

⇒∠2 (1 + ∠3) = 180°

⇒ ∠1 + ∠3 = (180°)/2 = 90°

∴ ∠R = 90°

In ∆PQR, by Pythagoras theorem,

PR² + QR² = PQ²