Given,

In ∆PQR,

N is a point on PR, such that QN ⊥ PR

And PN.NR = QN²

We have,

PN.NR = QN²

⇒ PN.NR = QN.QN

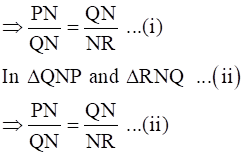
And

∠PNQ = ∠RNQ (common angle)

∴ ∆QNP ∼ ∆RNQ

Then, ∆QNP and ∆RNQ are equiangular.

∠PQN + ∠RQN = ∠QRN + ∠QPN

⇒ ∠PQR = ∠QRN + ∠QPN …..(ii)

We know that, sum of angles of a triangle = 180°

In ∆PQR,

∠PQR + ∠QPR + ∠QRP = 180°

⇒∠PQR + ∠QPN + ∠QRN = 180°

[∵ ∠QPR = ∠QPN and ∠QRP = ∠QRN]

⇒∠PQR + ∠PQR = 180°

⇒ 2∠PQR = 180°

[using Equation (ii)]

⇒ ∠PQR = 180°/2 = 90°

∴ ∠PQR = 90°

[each angle is equal to 90° by SAS similarity criteria]

Hence Proved.

Note:

To remember the process first, show that ∆QNP ∼ ∆RNQ, by SAS similarity criterion and then use the property that sum of all angles of a triangle is 180°.