Foot of a 10 m long ladder leaning against a vertical well is 6 m away from the base of the wall. Find the height of the point on the wall where the top of the ladder reaches.
Given,
Let PQ be a vertical wall and PR = 10m is a ladder.
The top of the ladder reaches to P and distance of ladder from the base of the wall QR is 6m.
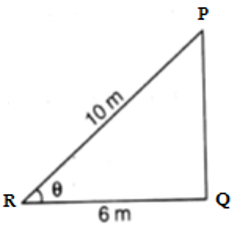
In right angled ∆PQR,
PR2 = PQ2 + QR2 [by Pythagoras theorem]
⇒ (10)2 = PQ2 + (6)2
⇒ 100 = PQ2 + 36
⇒ PQ2 = 100 – 36 = 64
∴ PQ = √64 = 8 cm
Hence, the height of the point on the wall where the top of the ladder reaches is 8cm.
16