Draw a ∆ABC in which BC = 6 cm, CA=5cm and AB=4cm. Construct triangle similar to it and of scale factor 5/3. Thinking process Here scale factor i.e., mn then the triangle to be constructed is larger than the given triangle. Use this concept and then constant the required triangle.

Question

Draw a ∆ABC in which BC = 6 cm, CA=5cm and AB=4cm. Construct triangle similar to it and of scale factor 5/3. Thinking process Here scale factor i.e., m>n then the triangle to be constructed is larger than the given triangle. Use this concept and then constant the required triangle.

Philoid · Accepted Answer

Steps of construction

1. Draw the line BC=6cm.

2. B and C as centers, draw two arcs of radius 4 cm and 5cm intersecting each other at A.

3. Join BA and CA. ∆ABC is the required triangle.

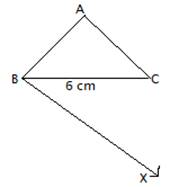
4. Draw any ray BX downwards making at acute angle.

5. Mark five points B₁, B_2, B₃, B₄ and B₅ on BX, such that

BB₁=B₁B₂=B₂B₃=B₃B₄=B₄B₅

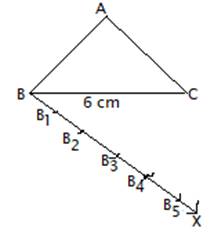
6. Join B₃C and draw B₅M||B₃C intersecting the extended line segment BC at M.

7. Draw MN||CA intersecting the extended line segment BA at N.

Then,

∆NBM is the required triangle whose sides is equal to 5/3 of the corresponding sides of the ∆ABC.

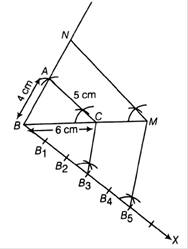
Hence, ∆NBM is the required triangle.

Draw a ∆ABC in which BC = 6 cm, CA=5cm and AB=4cm. Construct triangle similar to it and of scale factor 5/3.Thinking processHere scale factor i.e., m>n then the triangle to be constructed is larger than the given triangle. Use this concept and then constant the required triangle.

Draw a ∆ABC in which BC = 6 cm, CA=5cm and AB=4cm. Construct triangle similar to it and of scale factor 5/3.
Thinking process

Here scale factor i.e., m>n then the triangle to be constructed is larger than the given triangle. Use this concept and then constant the required triangle.