A solid right circular cone of height 120 cm and radius 60 cm is placed in a right circular cylinder full of water of height
180 cm. Such that it touches the bottom. Find the volume of water left in the cylinder, if the radius of the cylinder is equal to the radius to the cone.
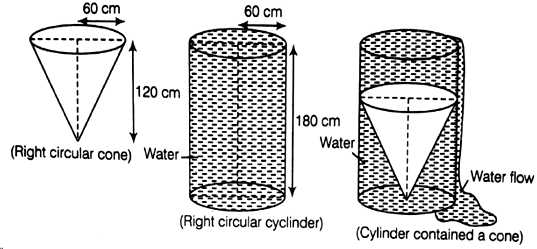
Whenever we placed a solid right circular cone in a right circular cylinder with full of water, then volume of a solid right circular cone is equal to the volume of water felled from the cylinder and Total volume of water in a cylinder is equal to the volume of the cylinder.
Therefore, we have,
Volume of water left in the cylinder = (Volume of cylinder) – (Volume of cone)
For cylinder,
Base radius, r = radius of cone = 60 cm
Height, h = 180 cm
As we know,
Volume of cylinder = πr2h
Where r is base radius and h is height of cylinder.
Volume of cylinder = π(60)2(180)
![]()
For cone
Base radius, r = 60 cm
Height, h = 120 cm
As we know,
![]()
Where r is base radius and h is height of cylinder.
![]()
So,
Volume of water left in cylinder = 2036571.43 - 452571.43 = 1584000 cm3