RS Aggarwal - Mathematics

Book: RS Aggarwal - Mathematics

Chapter: 12. Circles

Subject: Maths - Class 10th

Q. No. 15 of Exercise 12A

Listen NCERT Audio Books to boost your productivity and retention power by 2X.

15

In the given figure, a circle with center O, is inscribed in a quadrilateral ABCD such that it touches the side BC, AB, AD and CD at points P, Q, R and S respectively. If AB = 29 cm, AD = 23 cm, LB = 90° and DS = 5 cm then find the radius of the circle.

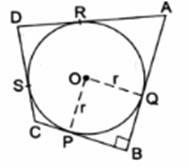
In quadrilateral POQB


∠OPB = 90°


[Tangent at any point on the circle is perpendicular to the radius through point of contact]


∠OQB = 90°


[Tangent at any point on the circle is perpendicular to the radius through point of contact]


∠PQB = 90° [Given]


By angle sum property of quadrilateral PQOB


∠OPB + ∠OQB + ∠PBQ + ∠POQ = 360°


90° + 90° + 90° + ∠POQ = 360°


∠POQ = 90°


As all angles of this quadrilaterals are 90° The quadrilateral is a rectangle


Also, OP = OQ = r


i.e. adjacent sides are equal, and we know that a rectangle with adjacent sides equal is a square


∴ POQB is a square


And OP = PB = BQ = OQ = r [1]


Now,


As we know that tangents drawn from an external point to a circle are equal


In given figure, We have


DS = DR = 5 cm


[Tangents from point D and DS = 5 cm is given]


AD = 23 cm [Given]


AR + DR = 23


AR + 5 = 23


AR = 18 cm


Now,


AR = AQ = 18 cm


[Tangents from point A]


AB = 29 cm [Given]


AQ + QB = 29


18 + QB = 29


QB = 11 cm


From [1]


QB = r = 11 cm


Hence Radius of circle is 11 cm.


Chapter Exercises

Exercise 12A
Exercise 12B
Multiple Choice Questions (MCQ)
Formative Assessment (Unit Test)

More Exercise Questions

1

Find the length of tangent drawn to a circle with radius 8 cm from a point 17 cm away from the center of the circle.
2

A point P is 25 cm away from the center of a circle and the length of tangent drawn from P to the circle is 24 cm. Find the radius of the circle.
3

Two concentric circles are of radii 6.5 cm and 2.5 cm. Find the length of the chord of the larger circle which touches the smaller circle.
4

In the given figure, a circle inscribed in a triangle ABC, touches the sides AB, BC and AC at points D, E and F respectively. If AB = 12 cm, BC = 8 cm and AC = 10 cm, find the lengths of AD, BE and CF.
5

In the given figure, PA and PB are the tangent segments to a circle with center 0. Show that the points A, O, B and P are concyclic.
6

In the given figure, the chord AB of the larger of the two concentric circles, with center O, touches the smaller circle at C. Prove that AC = CB.
7

From an external point P, tangents PA and PB are drawn to a circle with center O. If CD is the tangent to the circle at a point E and PA = 14 cm, find the perimeter of △PCD.
8

A circle is inscribed in a LABC touching AB, BC and AC at P, Q and R respectively. If AB = 10 cm, AR = 7 cm and CR = 5 cm, find the length of BC.
9

In the given figure, a circle touches all the four sides of a quadrilateral ABCD whose three sides are AB = 6 cm, BC = 7 cm and CD = 4 cm. Find AD.
10

In the given figure, an isosceles triangle ABC, with AB = AC, circumscribes a circle. Prove that the point of contact P bisects the base BC.
11

In the given figure, O is the center of two concentric circles of radii 4 cm and 6 cm respectively. PA and PB are tangents to the outer and inner circle respectively. If PA = 10 cm, find the length of PB up to one place of decimal.
12

In the given figure, a triangle ABC is drawn to circumscribe a circle of radius 3 cm such that the segments BD and DC into which BC is divided by the point of contact D, are of lengths 6 cm and 9 cm respectively. If the area of ABC = 54 cm2 then find the lengths of sides AB and AC.
13

PQ is a chord of length 4.8 cm of a circle of radius 3 cm. The tangents at P and Q intersect at a point T as shown in the figure. Find the length of TP.
14

Prove that the line segment joining the points of contact of two parallel tangents of a circle, passes through its center.
15

In the given figure, a circle with center O, is inscribed in a quadrilateral ABCD such that it touches the side BC, AB, AD and CD at points P, Q, R and S respectively. If AB = 29 cm, AD = 23 cm, LB = 90° and DS = 5 cm then find the radius of the circle.
16

In the given figure, O is the center of the circle and TP is the tangent to the circle from an external point T. If ∠PBT = 30°, prove that BA : AT = 2 : 1.

Students Store