Let sides AB, BC, CD, and AD touches circle at P, Q, R and S respectively.

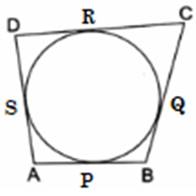
As we know that tangents drawn from an external point to a circle are equal ,

In the given image we have,

AP = AS = w (say) [Tangents from point A]

BP = BQ = x (say) [Tangents from point B]

CP = CR = y (say) [Tangents from point C]

DR = DS = z (say) [Tangents from point D]

Now,

Given,

AB = 6 cm

AP + BP = 6

w + x = 6 [1]

BC = 9 cm

BP + CP = 9

x + y = 9 [2]

CD = 8 cm

CR + DR = 8

y + z = 8 [3]

Also,

AD = AS + DS = w + z [4]

Add [1] and [3] and substracting [2] from the sum we get,

w + x + y + z - (x + y) = 6 + 8 - 9

w + z = 5 cm

From [4]

AD = 5 cm