In a circle of radius 7 cm, tangent PT is drawn from a point P such that PT = 24 cm. If O is the center of the circle, then length OP = ?
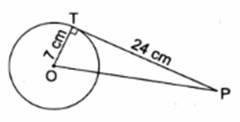
We have given, PT is a tangent drawn at point T on the circle.
∴ OT ⏊ TP
[Tangents drawn at a point on circle is perpendicular to the radius through point of contact]
So, In △OTP we have,
By Pythagoras Theorem,
[i.e. (Hypotenuse)2 = (Base)2 + (Perpendicular)2]
(OP)2 = (OT)2 + (PT)2
(OP)2 = (7)2 + (24)2
(OP)2 = 49 + 576
(OP)2 = 625
⇒ OP = 25 cm
3