PQ is a tangent to a circle with center O at the point P. If ΔOPQ is an isosceles triangle, then ∠ OQP is equal to

Question

Philoid · Accepted Answer

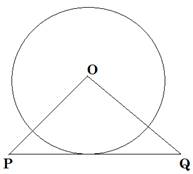
Let us consider a circle with center O and PQ is a tangent

on the circle, Joined OP and OQ

But OPQ is an isosceles triangle, ∴ OP = PQ

∠OQP = ∠POQ

[Angles opposite to equal sides are equal]

In △OQP

∠OQP + ∠OPQ + ∠POQ = 180°

[Angle sum property of triangle]

∠OQP + 90° + ∠OPQ = 180°

2 ∠OPQ = 90°

∠OPQ = 45°