In the given figure, O is the center of the circle. AB is the tangent to the circle at the point P. If ∠PAO = 30° then ∠CPB + ∠ACP is equal to

Question

In the given figure, O is the center of the circle. AB is the tangent to the circle at the point P. If ∠ PAO = 30° then ∠ CPB + ∠ ACP is equal to

Philoid · Accepted Answer

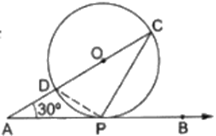
In given Figure, Join OP

In △OPC,

OP = OC [Radii of same circle]

∠OCP = ∠OPC

[Angles opposite to equal sides are equal]

∠ACP = ∠OPC

[As ∠OCP = ∠ACP] …[1]

Now,

∠OPB = 90°

[Tangents drawn at a point on circle is perpendicular to the radius through point of contact]

∠OPC + ∠CPB = 90°

∠ACP + ∠CPB = 90° [By 1]

So,

∠CPB + ∠ACP = 90°