In the given figure, LABC is right - angled at B such that BC = 6 cm and AB = 8 cm. A circle with center O has been inscribed inside the triangle. OP ⊥AB, OQ ⊥BC and OR ⊥AC. If OP = OQ = OR = x cm then x = ?
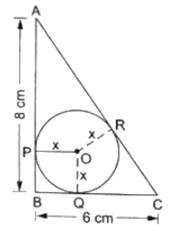
As ABC is a right triangle,
By Pythagoras Theorem
[i.e. (hypotenuse)2 = (perpendicular)2 + (base)2]
(AC)2 = (BC)2 + (AB)2
(AC)2 = (6)2 + (8)2
(AC)2 = 36 + 64 = 100
AC = 10 cm
Now,
ar(△ABC) = ar(△AOB) + ar(△BOC) + ar(△AOC)
As we know,
Area of triangle is 1/2 × Base × Height(Altitude)
Now,
OP ⏊ AB [Given]
∴ OP is the altitude in △AOB
OQ ⏊ BC [Given]
∴ OQ is the altitude in △BOC
OR ⏊ AC [Given]
∴ OR is the altitude in △AOC
So, we have
1/2 × BC × AB = (1/2 × AB × OP) + (1/2 × BC × OQ) + (1/2 × AC × OR)
6(8) = 8(x) + 6(x) + 10(x)
[∵ OP = OQ = OR = x, Given]
48 = 24x
x = 2 cm