If two tangents are drawn to a circle from an external point, show that they subtend equal angles at the center.

Question

Philoid · Accepted Answer

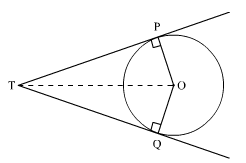
Let PT and PQ are two tangents from external point P to a circle with center O

To Prove : PT and PQ subtends equal angles at center i.e. ∠POT = ∠QOT

In △OPT and △OQT

OP = OQ [radii of same circle]

OT = OT [common]

PT = PQ [Tangents drawn from an external point are equal]

△OPT ≅ △OQT [By Side - Side - Side Criterion]

∠POT = ∠QOT [Corresponding parts of congruent triangles are congruent]

Hence, Proved.