Let us assume f (x) = x⁴ + 4x³ ‒ 2x² ‒ 20x - 15

As (x-√5) and (x-√5) are the zeros of the given polynomial therefore each one of (x-√5) and (x + √5) is a factor of f (x)

Consequently, (x-√5) (x + √5) = (x² – 5) is a factor of f (x)

Now, on dividing f (x) by (x² – 5) we get:

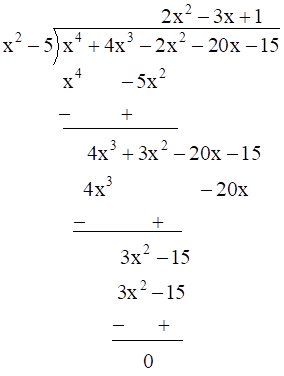
f (x) = 0

x⁴ + 4x³ – 7x² – 20x – 15 = 0

(x² – 5) (x² + 4x + 3) = 0

(x -√5) (x + √5) (x + 1) (x + 3) = 0

∴ x = √5 or x = - √5 or x = - 1 or x = - 3

Hence, all the zeros of the given polynomial are √5, -√5, - 1 and - 3