Let 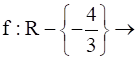R be a function defined as 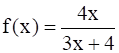. The inverse of f is the map g : Range 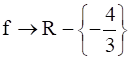given by

Question

Philoid · Accepted Answer

It is given that R be a function defined as f (x) = .

Let y be any element of Range f.

Then, there exists x ϵ R - such that y = f(x)

⟹ 3xy + 4y = 4x

⟹ x(4 – 3y) = 4y

⟹ x =

Let us define g: Range f → R - as g(y) =

Now, (gof)(x) = g(f(x)) =

And, (fog)(y) = f(g(y)) =

Therefore, gof = and fog = I_{Range f}

Thus, g is the inverse of f

Therefore, The inverse of f is the map

: Range f → R - , which is given by g(y) =