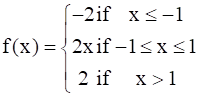
The given function is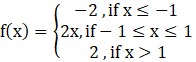

The function f is defined at all points of the real line.

Then, we have 5 cases i.e., k < -1, k = -1, -1 < k < 1, k = 1 or k > 1.

Now, Case I: k < 0

Then, f(k) = -2

= -2= f(k)

Thus,

Hence, f is continuous at all points x, s.t. x < -1.

Case II: k = -1

f(k) = f(=1) = -2

= -2

= 2 × (-1) = -2

Hence, f is continuous at x = -1.

Case III: -1 < k < 1

Then, f(k) = 2k

= 2k = f(k)

Thus,

Hence, f is continuous in (-1, 1).

Case IV: k = 1

Then f(k) = f(1) = 2 × 1 = 2

= 2 × 1 = 2

= 2

Hence, f is continuous at x = 1.

Case V: k > 1

Then, f(k) = 2

= 2 = f(k)

Thus,

Hence, f is continuous at all points x, s.t. x > 1.

Therefore, f is continuous at all points of the real line.