It is given function is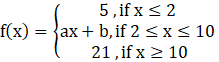

We know that the given function f is defined at all points of the real line.

Thus, f is continuous at x = 2, we get,

⇒

⇒ 5 = 2a + b = 5

⇒ 2a + b = 5………………(1)

Thus, f is continuous at x = 10, we get,

⇒

⇒ 10a + b = 21 =21

⇒ 10a + b = 21………………(2)

On subtracting eq. (1) from eq. (2), we get,

8a = 16

⇒ a = 2

Thus, putting a = 2 in eq. (1), we get,

2 × 2 + b = 5

⇒ 4 + b = 5

⇒ b = 1

Therefore, the values of a and b for which f is a continuous function are 2 and 1 resp.