If A =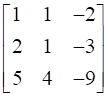 , find |A|.
, find |A|.
GIVEN: 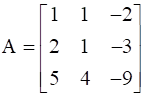
Now, 
We know that a determinant of a 3 x 3 matrix is calculated as
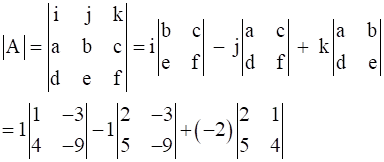 .
.
= 1[-9 – (-3)(4)] – 1[2(-9) – (-3)(5)] – 2[2(4) – 1(5)]
= 1[-9 + 12] – 1[-18 + 15] – 2[8 – 5]
= 1[3] – 1[-3] – 2[3]
= 3 + 3 – 6
= 0
Ans. |A| = 0
11
If A =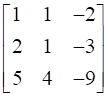 , find |A|.
, find |A|.
GIVEN: 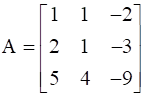
Now, 
We know that a determinant of a 3 x 3 matrix is calculated as
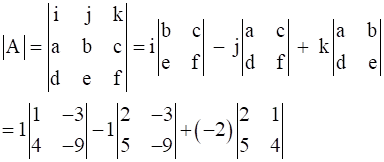 .
.
= 1[-9 – (-3)(4)] – 1[2(-9) – (-3)(5)] – 2[2(4) – 1(5)]
= 1[-9 + 12] – 1[-18 + 15] – 2[8 – 5]
= 1[3] – 1[-3] – 2[3]
= 3 + 3 – 6
= 0
Ans. |A| = 0