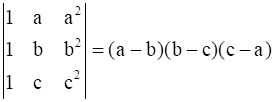
R1 → R1 - R2 (i.e. Replacing 1st row by subtraction of 1st and 2nd row)
R2 → R2 - R3 (i.e. Replacing 2nd row by subtraction of 2nd and 3rd row)
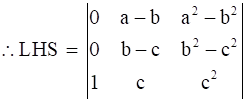
Since we know a2 - b2 = (a + b)(a - b)
Therefore taking (a - b) and (b - c) outside the determinant from 1st and 2nd row respectively

R1 → R1 - R2 (i.e. Replacing 1st row by subtraction of 1st and 2nd row)
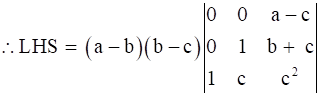
Expanding the determinant along 1st column
∴
∴LHS = (a - b)(b - c)(0 - (a - c))
∴LHS = (a - b)(b - c)(c - a) = RHS
∴
13