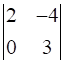
Minor: Minor of an element a_ij of a determinant is the determinant obtained by removing i^th row and j^th column in which element a_ij lies. It is denoted by M_ij.

Cofactor: Cofactor of an element a_ij, A_ij = (-1)^i+j M_ij.

Minor of element a_ij = M_ij

a₁₁ = 2, Minor of element a₁₁ = M₁₁ = 3

Here removing 1^st row and 1^st column from the determinant we are left out with 3 so M₁₁ = 3.

Similarly, finding other Minors of the determinant

a₁₂ = -4, Minor of element a₁₂ = M₁₂ = 0

a₂₁ = 0, Minor of element a₂₁ = M₂₁ = -4

a₂₂ = 3, Minor of element a₂₂ = M₂₂ = 2

Cofactor of an element a_ij, A_ij = (-1)^i+j × M_ij

A₁₁ = (-1)¹⁺¹ × M₁₁ = 1 × 3 = 3

A₁₂ = (-1)¹⁺² × M₁₂ = (-1) × 0 = 0

A₂₁ = (-1)²⁺¹ × M₁₁ = (-1) × (-4) = 4

A₂₂ = (-1)²⁺² × M₂₂ = 1 × 2 = 2