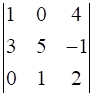
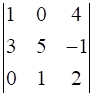
Minor of an element a_ij = M_{ij �}

a₁₁ = 1, Minor of element a₁₁ = M₁₁ = 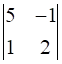= (5 × 2) – ((-1) × 1) = 10 + 1 = 11

Here removing 1^st row and 1^st column from the determinant we are left out with the determinant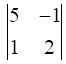. Solving this we get M₁₁ = 11

Similarly, finding other Minors of the determinant

a₁₂ = 0, Minor of element a₁₂ = M₁₂ = 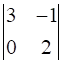= (3 × 2) – ((-1) × 0) = (6 - 0) = 6

a₁₃ = 4, Minor of element a₁₃ = M₁₃ = 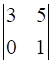= (3 × 1) – (5 × 0) = 3 - 0 = 3

a₂₁ = 3, Minor of element a₂₁ = M₂₁ = 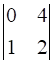= (0 × 2) – (4 × 1) = 0 – 4 = -4

a₂₂ = 5, Minor of element a₂₂ = M₂₂ = 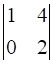= (1 × 2) – (4 × 0) = 2 – 0 = 2

a₂₃ = -1, Minor of element a₂₃ = M₂₃ = = (1 × 1) – (0 × 0) = 1

a₃₁ = 0, Minor of element a₃₁ = M₃₁ = 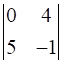= (0 × (-1)) – (4 × 5) = 0 – 20 = -20

a₃₂ = 1, Minor of element a₃₂ = M₃₂ = 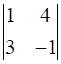= (1 × (-1)) – (4 × 3) = -1 – 12 = -13

a₃₃ = 2, Minor of element a₃₃ = M₃₃ = = (1 × 5) – (0 × 3) = (5 – 0) = 5

Cofactor of an element a_ij, A_ij = (-1)^i+j × M_ij

A₁₁ = (-1)¹⁺¹× M₁₁ = 1 × 11 = 11

A₁₂ = (-1)¹⁺²× M₁₂ = (-1) × 6 = -6

A₁₃ = (-1)¹⁺³× M₁₃ = 1 × 3 = 3

A₂₁ = (-1)²⁺¹× M₂₁ = (-1) × (-4) = 4

A₂₂ = (-1)²⁺² × M₂₂ = 1 × 2 = 2

A₂₃ = (-1)²⁺³ × M₂₃ = (-1) × 1 = -1

A₃₁ = (-1)³⁺¹ × M₃₁ = 1 × (-20) = -20

A₃₂ = (-1)³⁺² × M₃₂ = (-1) × (-13) = 13

A₃₃ = (-1)³⁺³ × M₃₃ = 1 × 5 = 5