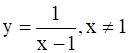
It is given that equation of the curve y =

Now, slope of the tangent to the given curve at a point (x, y) is:

Now, if the slope of the tangent is -1, then we get,

⇒ (x-1)² = 1

⇒ (x-1) = 1

⇒ x = 2, 0

So, when x = 2 then y = 1

And when x = 0 then y = 1

Therefore, required points are (0, -1) and (2, 1).

Now, the equation of the tangent (0,1) is given by:

y – (-1) = -1(x-0)

⇒ y + 1 = -x

⇒ y + x+ 1 = 0

And the equation of the tangent (2,1) is given by:

y – 1 = -1(x-2)

⇒ y - 1 = -x +2

⇒ y + x - 3 = 0

Therefore, the equations of the required lines are y + x+ 1 = 0 and y + x - 3 = 0.