Find the intervals in which the function f given by 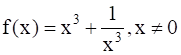 is
is
(i) Increasing (ii) decreasing.
It is given that f(x) =![]()
![]()
Then, f’(x) =0
⇒ 3x6 - 3 = 0
⇒ x6 = 1
⇒ x = ![]() 1
1
Now, the points x =1 and x = - 1 divide the real line into three disjoint intervals
( - ∞, - 1), ( - 1,1) and (1,∞).
In interval ( - ∞, - 1) and (1,∞) when x < - 1 and x > 1 then f’(x) >0
Therefore, when x < - 1 and x > 1, f is increasing.
And, in interval ( - 1,1) when - 1< x < 1 then f’(x) < 0.
Therefore, when - 1 < x < 1, f is decreasing.
7