It is given that ellipse

Let the major axis be along the x – axis.1).

Let ABC be the triangle inscribed in the ellipse where vertex C is at (a,0).

Since, the ellipse is symmetrical w.r.t. x - axis and y - axis, we can assume the coordinates of A to be ( - x₁,y₁) and the coordinates of B to be ( - x₁, - y₁).

Now, we have y1 = ± 

Therefore, Coordinates of A and the coordinates of B

As the point(x₁,y₁) lies on the ellipse, the area of triangle ABC (A) is given by:

A =

……..(1)

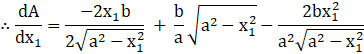
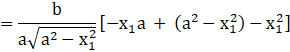
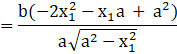
Now,

But, x₁ cannot be equal to a.

⇒ x₁ =

y₁ =

Now,

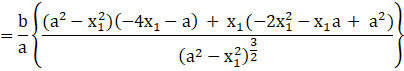
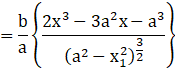
Also, when x₁ = , then,

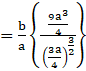< 0

Then, the area is the maximum when x₁ = .

Therefore, Maximum area of the triangle is given by:

A =