Given,

Applying elementary transformations we get,

R₁→ R₁ + R₂ + R₃

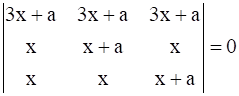
⇒ (3x + a) 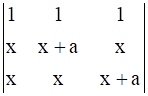= 0

C₂→ C₂ – C₁ and C₃→ C₃ – C₁, we get

(3x + a) 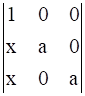= 0

Expanding along R₁ we have

(3x + a) [1 (a × a – 0 × 0) – 0 (x × a – 0 × a) + 0 (x × 0 – a × x)] = 0

⇒ (3x + a) [1 × a²] = 0

⇒ a² (3x + a) = 0

Since, a ≠ 0

∴ 3x + a = 0

⇒ x = -a/3