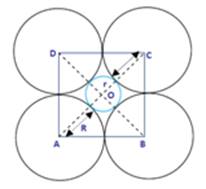
In the given figure, let the sphere have the centre, O and is fitted in the octahedral void.

As given, radius of the sphere fitted in the octahedral void = r

And the radius of the atoms in close packing = R

Here, angle AOD = 90⁰

In triangle AOD,

DA² = OA² + OD²

⇒ (R+R)² = ( R + r )²+ (R+r)²

⇒ 4R² = 2(R+r)²

⇒ 2R² = (R+r)²

⇒ √2 R = (R+r)

⇒ R + r = R

⇒ r = R – R

⇒ r = R (1.414 - 1)

⇒ r = 0.414 R

This is the required relation between r and R.