Of all the closed cylindrical cans (right circular), of a given volume of 100 cubic centimetres, find the dimensions of the can which has the minimum surface area?

Question

Philoid · Accepted Answer

Let r be the radius and h be the height of the cylinder.

Let V be the volume of the cylinder. Then

V = πr²h = 100(given)

⇒ h =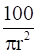

hen, the surface area (S) of the cylinder is given by:

S = 2πr² + 2πrh

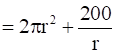
Now, 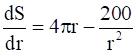, 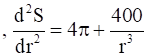<0

If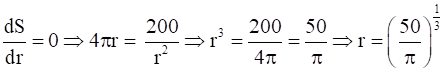

So, w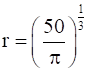hen then 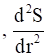> 0

Then, by second derivative test, the surface area is the minimum when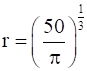

Now, when 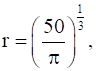then h = 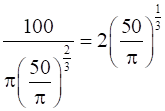cm.

Therefore, the dimensions of the can which has the minimum surface area are 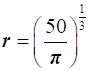and h cm.