If 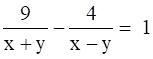 and
and 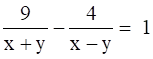 then
then
We have,
![]() …(i)
…(i)
![]() …(ii)
…(ii)
Now, substituting ![]() and
and ![]() in (i) and (ii) we get:
in (i) and (ii) we get:
3u + 2v = 2 …(iii)
9u – 4v = 1 …(iv)
Multiplying (iii) by 2 and adding it with (iv) we get:
6u + 9u = 4 + 1
![]()
![]()
Multiplying again (iii) by 2 and then subtracting it from (iv), we get:
6v + 4v = 6 – 1
![]()
![]()
∴ x + y = 3 …(v)
And, x – y = 2 …(vi)
Now, by adding (v) and (vi) we get:
2x = 3 + 2
![]()
Substituting the value of x in (v), we get
![]()
![]()
![]()
![]()
Hence, option B is correct
6