Book: RS Aggarwal - Mathematics
Chapter: 18. Area of Circle, Sector and Segment
Subject: Maths - Class 10th
Q. No. 5 of Exercise 18B
Listen NCERT Audio Books to boost your productivity and retention power by 2X.
A wire when bent in the form of an equilateral triangle endoses an area of 121√3 cm2. The same wire is bent to form a circle. Find the area enclosed by the circle.
In this question the wire is first bent in the shape of equilateral triangle and then same wire is bent to form a circle. The point to be noticed is that the same wire is used both the times which implies that the perimeter of equilateral triangle and that of circle will be equal.
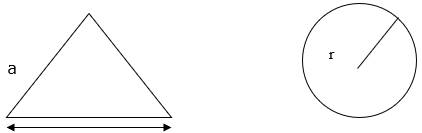
Let the equilateral triangle be of side ‘a’ cm and radius of the circle be ‘r’.
Given: Area enclosed by equilateral triangle = 123√3 cm2
Also, we know that Area of equilateral triangle
Where ‘a’ = side of equilateral triangle
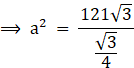
⟹ a = √484
⇒ a = 22 cm
Therefore, side of equilateral triangle, ‘a’ is 22 cm.
Also, circumference of the circle = Perimeter of equilateral triangle → eqn1
Perimeter of equilateral triangle = 3×side
= 3×22
= 66 cm → eqn2
Also, we know Circumference of circle = 2πr → eqn3
Put values in equation 1 from equation 2 & 3, we get
2πr = 66
(put π = 22/7)
On rearranging,
⇒ r = 10.5 cm
So, the radius ‘r’ of the circle is 10.5 cm.
Area of circle = πr2
Where r = radius of the circle
⇒ Area of circle = π(10.52)
= 346.5 cm2
Area of the circle is 346.5 cm2.
