Find the area enclosed between the parabola y2 = 4ax and the line y = mx.
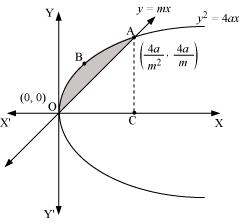
We can see from the figure that the area of the region bounded by the curve y2 = 4ax and the line y = mx is shown by shaded region that is Area OABO.
The points of intersection of both the curves are (0,0) and 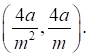
Now draw AC perpendicular to x – axis.
Thus,
Area of OABO = Area OCABO – Area (ΔOCA)
⇒ 
⇒ 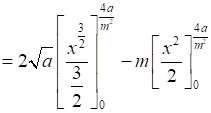
⇒ 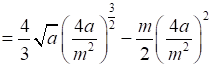
⇒ 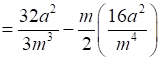
⇒ 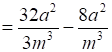
⇒ 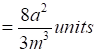
6