The Cartesian equation of a line is 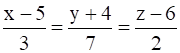 . Write its vector form.
. Write its vector form.
We know that
The Cartesian equation of a line through a point (x1, y1, z1) and having direction cosines l, m, n is 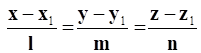 .
.
Comparing this standard form with the given equation, we get
x1 = 5, y1 = -4, z1 = 6 and l = 3, m = 7, n = 2
⇒ The point through which the line passes has the position vector ![]() and the vector parallel to the line is given by
and the vector parallel to the line is given by ![]() .
.
Now, ∵ Vector equation of a line that passes through a given point whose position vector is ![]() and parallel to a given vector
and parallel to a given vector ![]() is
is ![]() .
.
∴ The vector equation of the required line is:
⇒ ![]()
8