For each of the differential equations in question, find the general solution:

Question

Philoid · Accepted Answer

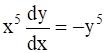
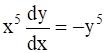
Separating variables,

Or,

Integrating both sides,

Letbe a constant,

Or,

0r,