In each of the question, show that the given differential equation is homogeneous and solve each of them.

Question

In each of the question, show that the given differential equation is homogeneous and solve each of them. (x – y)dy – (x + y)dx = 0

Philoid · Accepted Answer

(x - y)dy = (x + y)dx

Here, putting x = kx and y = ky

= k⁰.f(x,y)

Therefore, the given differential equation is homogeneous.

(x - y)dy – (x + y)dx = 0

To make it we make the substitution.

y = vx

Differentiating eq. with respect to x, we get

Integrating both sides we get,

2vdv = dt

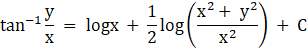
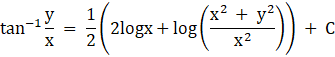
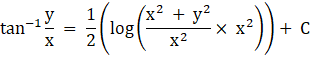
The required solution of the differential equation.