A homogeneous differential equation of the from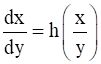 
can be solved by making the substitution.
Therefore, we shall substitute,
x = vy
16
A homogeneous differential equation of the from 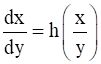can be solved by making the substitution.
Therefore, we shall substitute,
x = vy