Mathematics Part-II

Book: Mathematics Part-II

Chapter: 9. Differential Equations

Subject: Maths - Class 12th

Q. No. 15 of Exercise 9.6

Listen NCERT Audio Books - Kitabein Ab Bolengi

15

For each of the differential equations given in question, find a particular solution satisfying the given condition:

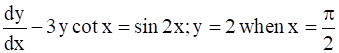
It is given that

This is equation in the form of (where, p = -3cotx and Q = sin2x)


Now, I.F. =


Thus, the solution of the given differential equation is given by the relation:


y(I.F.) =




⇒ y cosec3x = 2cosecx + C


⇒ y =


⇒ y = -2sin2x + Csin3x--------------(1)


Now, it is given that y = 2 when x =


Thus, we get,


2 = -2 + C


⇒ C = 4


Now, Substituting the value of C = 4 in (1), we get,


y = -2sin2x + 4sin3x


⇒ y = 4sin3x - 2sin2x


Therefore, the required general solution of the given differential equation is


y = 4sin3x - 2sin2x.


15

Chapter Exercises

Exercise 9.1
Exercise 9.2
Exercise 9.3
Exercise 9.4
Exercise 9.5
Exercise 9.6
Miscellaneous Exercise

More Exercise Questions

1

For each of the differential equations given in question, find the general solution:
2

For each of the differential equations given in question, find the general solution:
3

For each of the differential equations given in question, find the general solution:
4

For each of the differential equations given in question, find the general solution:
5

For each of the differential equations given in question, find the general solution:
6

For each of the differential equations given in question, find the general solution:
7

For each of the differential equations given in question, find the general solution:
8

For each of the differential equations given in question, find the general solution:

(1 + x2)dy + 2xy dx = cot x dx (x ≠ 0)
9

For each of the differential equations given in question, find the general solution:
10

For each of the differential equations given in question, find the general solution:
11

For each of the differential equations given in question, find the general solution:

y dx + (x – y2)dy = 0
12

For each of the differential equations given in question, find the general solution:
13

For each of the differential equations given in question, find a particular solution satisfying the given condition:
14

For each of the differential equations given in question, find a particular solution satisfying the given condition:
15

For each of the differential equations given in question, find a particular solution satisfying the given condition:
16

Find the equation of a curve passing through the origin given that the slope of the tangent to the curve at any point (x, y) is equal to the sum of the coordinates of the point.
17

Find the equation of a curve passing through the point (0, 2) given that the sum of the coordinates of any point on the curve exceeds the magnitude of the slope of the tangent to the curve at that point by 5.
18

The Integrating Factor of the differential equation is
19

The Integrating Factor of the differential equation

is