Find the equation of the curve passing through the point 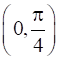whose differential equation is sin x cos y dx + cos x sin y dy = 0.

Mathematics Part-II

Book: Mathematics Part-II

Chapter: 9. Differential Equations

Subject: Maths - Class 12^th

Q. No. 8 of Miscellaneous Exercise

Listen NCERT Audio Books - Kitabein Ab Bolengi

8

Find the equation of the curve passing through the point whose differential equation is sin x cos y dx + cos x sin y dy = 0.

It is given that sin x cos y dx + cos x sin y dy = 0

⇒ tanxdx + tanydy = 0

So, on integrating both sides, we get,

log(secx) + log(secy) = logC

⇒ log(secx.secy) = log C

⇒ secx.secy = C

The curve passes through point

Thus, 1× = C

⇒ C =

On substituting C = in equation (1), we get,

secx.secy =

Therefore, the required equation of the curve is

8

Chapter Exercises

Exercise 9.1

Exercise 9.2

Exercise 9.3

Exercise 9.4

Exercise 9.5

Exercise 9.6

Miscellaneous Exercise

1

For each of the differential equations given below, indicate its order and degree (if defined).

1

For each of the differential equations given below, indicate its order and degree (if defined).

1

For each of the differential equations given below, indicate its order and degree (if defined).

2

For each of the exercises given below, verify that the given function (implicit or explicit) is a solution of the corresponding differential equation.
xy = a e^x + b e^–x + x² :

2

For each of the exercises given below, verify that the given function (implicit or explicit) is a solution of the corresponding differential equation.
y = e^x (a cos x + b sin x) :

2

For each of the exercises given below, verify that the given function (implicit or explicit) is a solution of the corresponding differential equation.
y = x sin 3x :

2

For each of the exercises given below, verify that the given function (implicit or explicit) is a solution of the corresponding differential equation.
x² = 2y² log y :

3

Form the differential equation representing the family of curves given by (x – a)² + 2y² = a², where a is an arbitrary constant.

4

Prove that x² – y² = c (x² + y²)² is the general solution of differential equation (x³–3xy²) dx = (y³–3x²y)dy, where c is a parameter.

5

Form the differential equation of the family of circles in the first quadrant which touch the coordinate axes.

6

Find the general solution of the differential equation

7

Show that the general solution of the differential equation is given by (x + y + 1) = A (1 – x – y – 2xy), where A is parameter.

8

Find the equation of the curve passing through the point whose differential equation is sin x cos y dx + cos x sin y dy = 0.

9

Find the particular solution of the differential equation (1 + e^2x) dy + (1 + y²) e^x dx = 0, given that y = 1 when x = 0.

10

Solve the differential equation

11

Find a particular solution of the differential equation (x – y) (dx + dy) = dx – dy, given that y = –1, when x = 0. (Hint: put x – y = t)

12

Solve the differential equation

13

Find a particular solution of the differential equation (x ≠ 0), given that y = 0 when

14

Find a particular solution of the differential equation , given that y = 0 when x = 0.

15

The population of a village increases continuously at the rate proportional to the number of its inhabitants present at any time. If the population of the village was 20, 000 in 1999 and 25000 in the year 2004, what will be the population of the village in 2009?

16

The general solution of the differential equation is

17

The general solution of a differential equation of the type is

18

The general solution of the differential equation e^x dy + (y e^x + 2x) dx = 0 is